.png)
Chapter –6 The Triangle and its Properties Triangles: A closed plane figure bounded by three linesegments. The six elements of a triangle are its three angles and thethree sides. The line segment joining a vertex of a triangle to the mid point of its opposite side is called a medianof the triangle. A triangle has3 medians. The perpendicular line segment froma vertex ofa triangle toits opposite sideis called analtitude of the triangle. A triangle has3 altitudes. Type oftriangle based onSides: Equilateral: A triangle is said to be equilateral, if each one of its sides has the same length. Inan equilateral triangle, each angle hasmeasure 60°. Isosceles: A triangle is said to be isosceles, if atleast any two of its sides are of same length. The non-equal side of an isosceles triangle is called its base; the base angles of an isosceles triangle have equal measure. Scalene: A triangle having all side of different lengths. It has no two angles equal. Property of thelengths of sides of a triangle: The sum ofthe lengths ofany two sides of a triangle is greater thanthe length ofthe third side. The difference between the lengths of any two sides is smaller than the length of the third side. This property is useful to know if it is possible to draw a triangle when the lengths ofthe three sides are known. Types of Triangle based on Angles: (i) Right Angled Triangle: A triangle one of whoseangles measures (ii) Obtused Angled Triangle: A triangle one of whose angles measures morethan (iii) Acute Angled Triangle: A triangle each of whoseangles measures lessthan In a rightangled triangle, the side opposite to the rightangle is calledthe hypotenuse andthe other twosides are called its legs. Pythagoras property: In a right-angled triangle, the square on the hypotenuse = the sumof the squares on its legs.If a triangle is not right-angled, this property doesnot hold good. Thisproperty is useful to decide whether a given triangle is right-angled
or not. An exterior angle of a triangle is formed, when a side of a triangle is produced. At each vertex, you have twoways of forming an exterior angle. A property of exterior angles: The measure of any exterior angle of a triangle is equal to the sumof the measures of its interior opposite angles. The angle sum property of a triangle: The total measure of the three angles of a triangle is180°. Property of the Lengths of Sides of a Triangle: The sum of the lengths of any two sides of a triangle is always greater than the length of the third side. The difference of the lengths of any twosides of a triangle is always smaller than the length of the third side.
Class 7Important Formulas Chapter 6 – TheTriangles and its Properties 1. A triangle is a figure madeup by three line segments joining, in pairs, three non-collinear points. That is, if A, B, C are three non-collinear points, the figure formed by three line segments AB,BC and CA iscalled a triangle with vertices A, B, C. 2. The three line segments forming a triangle are called thesides of thetriangle. 3. The three sides and three angles of a triangle aretogether called the sixparts or elements ofthe triangle. 4. A triangle whose twosides are equal, is called anisosceles triangle. 5. A triangle whose allsides are equal, is called anequilateral triangle. 6. A triangle whose notwo sides are equal, is called a scalene triangle. 7. A triangle whose all the angles are acute is called an acute triangle. 8. A triangle whose oneof the angles is a rightangle is called a right triangle. 9. A triangle whose one of the angles isan obtuse angle is called an obtuse triangle. 10. The interior of a triangle is made upof all such points P of theplane, as areenclosed by thetriangle. 11. The exterior of a triangle is that part of the plane which consists of thosepoints Q, which are neither on the triangle nor in its interior. 12. The interior of a triangle together with the triangle itself is called thetriangular region. 13. The sum of the angles of a triangle is two right angles or 180°. 14. If a side of a triangle is produced, theexterior angle so formed is equal to the sumof the interior opposite angles. 15. In any triangle, anexterior angle isgreater than either of the interior opposite angles. 16. The sum of anytwo sides of a triangle is greater thanthe third side. 17. In a right triangle, if a, b are the lengths ofthe sides and c that ofthe hypotenuse, then
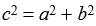
18. If the sides of a triangle are of lengths a, b and c such that
then the triangle is right-angled and the side of length c is the hypotenuse. 19. Three positive numbers a, b, c inthis order aresaid to form a Pythagorean triplet, if
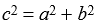
Triplets (3, 4, 5) (5, 12,13), (8, 15, 17), (7,24, 25) and (12, 35,37) are somePythagorean triplets.
ความคิดเห็น